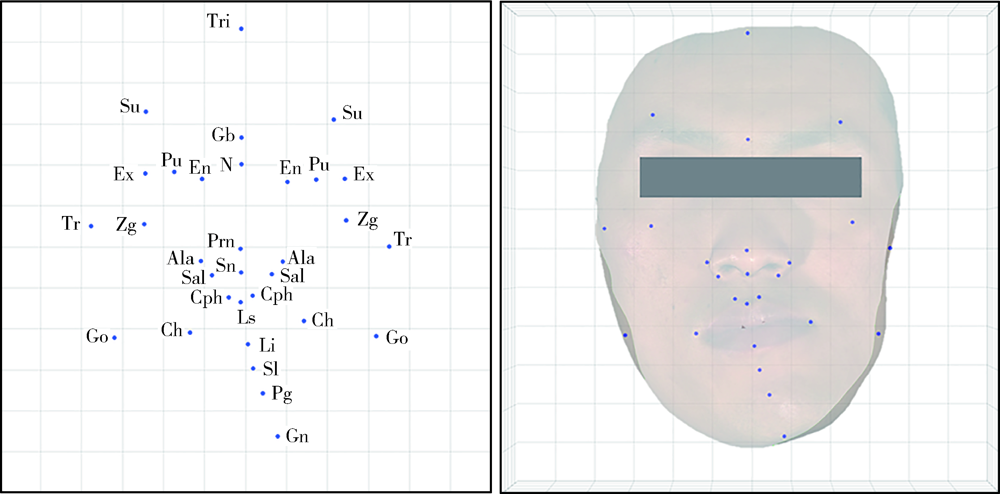
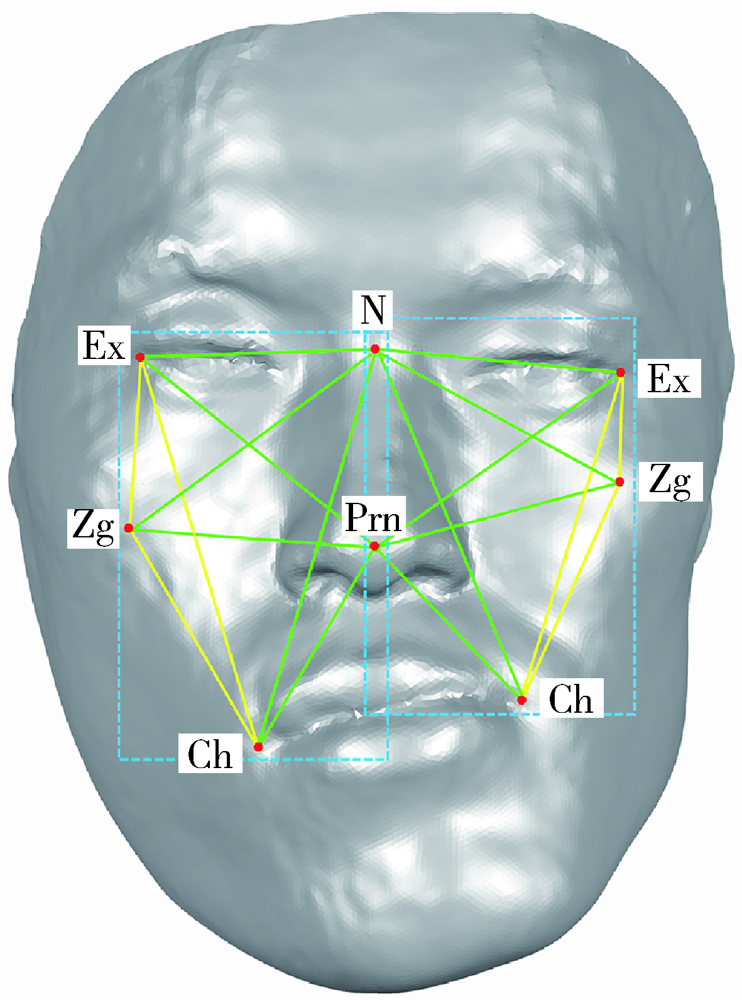
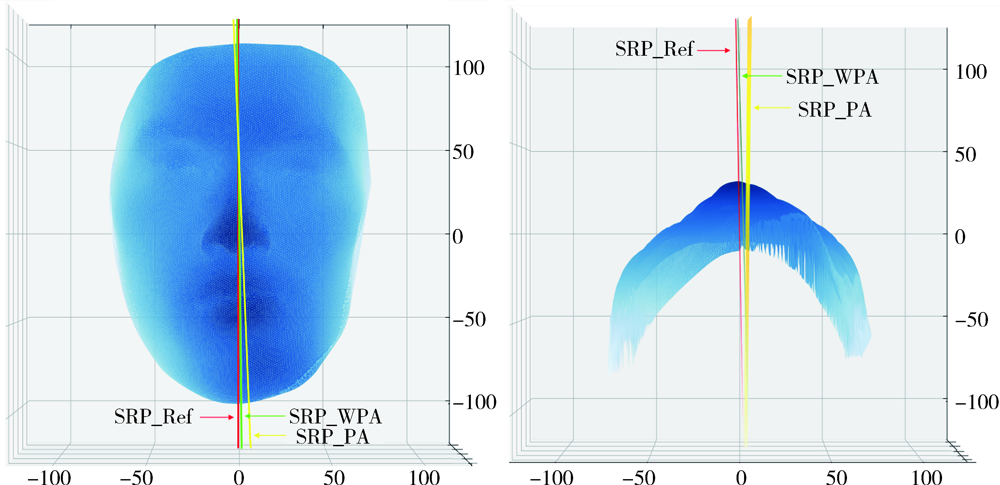
Journal of Peking University (Health Sciences) ›› 2021, Vol. 53 ›› Issue (1): 220-226. doi: 10.19723/j.issn.1671-167X.2021.01.034
Previous Articles Next Articles
A method for constructing three-dimensional face symmetry reference plane based on weighted shape analysis algorithm
ZHU Yu-jia1,2,ZHAO Yi-jiao1,2,ZHENG Sheng-wen3,4,WEN Ao-nan1,2,FU Xiang-ling3,4,Δ( ),WANG Yong1,2,Δ(
),WANG Yong1,2,Δ( )
)
- 1. Center of Digital Dentistry, Peking University School and Hospital of Stomatology & National Clinical Research Center for Oral Diseases & National Engineering Laboratory for Digital and Material Technology of Stomatology & Beijing Key Laboratory of Digital Stomatology, Beijing 100081, China
2. Department of Prosthodontics, Peking University School and Hospital of Stomatology & National Clinical Research Center for Oral Diseases & National Engineering Laboratory for Digital and Material Technology of Stomatology & Beijing Key Laboratory of Digital Stomatology, Beijing 100081, China
3. School of Computer Science, Beijing University of Posts and Telecommunications (National Pilot Software Engineering School), Beijing 100876, China
4. Key Laboratory of Trustworthy Distributed Computing and Service, Ministry of Education, Beijing University of Posts and Telecommunications, Beijing 100876, China
CLC Number:
- R782.2
| [1] |
Baudouin JY, Tiberghien G. Symmetry, averageness, and feature size in the facial attractiveness of women[J]. Acta Psychol (Amst), 2004,117(3):313-332.
doi: 10.1016/j.actpsy.2004.07.002 |
| [2] |
Ferrario VF, Sforza C, Ciusa V, et al. The effect of sex and age on facial asymmetry in healthy subjects: a cross-sectional study from adolescence to mid-adulthood[J]. J Oral Maxillofac Surg, 2001,59(4):382-388.
doi: 10.1053/joms.2001.21872 pmid: 11289167 |
| [3] |
Shaner DJ, Peterson AE, Beattie OB, et al. Assessment of soft tissue facial asymmetry in medically normal and syndrome-affected individuals by analysis of landmarks and measurements[J]. Am J Med Genet, 2000,93(2):143-154.
doi: 10.1002/1096-8628(20000717)93:2<143::aid-ajmg12>3.0.co;2-q pmid: 10869118 |
| [4] |
Burke PH, Healy MJ. A serial study of normal facial asymmetry in monozygotic twins[J]. Ann Hum Biol, 1993,20(6):527-534.
doi: 10.1080/03014469300002932 pmid: 8257078 |
| [5] | 黄佳梦, 李娟, 林军. 面部不对称畸形评估的研究进展[J]. 中国医疗美容, 2019,9(6):121-128. |
| [6] |
Kim MS, Lee EJ, Song IJ, et al. The location of midfacial landmarks according to the method of establishing the midsagittal reference plane in three-dimensional computed tomography analysis of facial asymmetry[J]. Imaging Sci Dent, 2015,45(4):227-232.
doi: 10.5624/isd.2015.45.4.227 pmid: 26730370 |
| [7] |
Lee MS, Chung DH, Lee JW, et al. Assessing soft-tissue characteristics of facial asymmetry with photographs[J]. Am J Orthod Dentofacial Orthop, 2010,138(1):23-31.
doi: 10.1016/j.ajodo.2008.08.029 pmid: 20620830 |
| [8] |
Haraguchi S, Iguchi Y, Takada K. Asymmetry of the face in orthodontic patients[J]. Angle Orthod, 2008,78(3):421-426.
doi: 10.2319/022107-85.1 pmid: 18416611 |
| [9] |
O’Grady KF, Antonyshyn OM. Facial asymmetry: three-dimensional analysis using laser surface scanning[J]. Plast Reconstr Surg, 1999,104(4):928-937.
doi: 10.1097/00006534-199909040-00006 pmid: 10654730 |
| [10] |
Nur RB, Cakan DG, Arun T. Evaluation of facial hard and soft tissue asymmetry using cone-beam computed tomography[J]. Am J Orthod Dentofacial Orthop, 2016,149(2):225-237.
doi: 10.1016/j.ajodo.2015.07.038 pmid: 26827979 |
| [11] |
Lee JK, Jung PK, Moon CH. Three-dimensional cone beam computed tomographic image reorientation using soft tissues as reference for facial asymmetry diagnosis[J]. Angle Orthod, 2014,84(1):38-47.
doi: 10.2319/112112-890.1 pmid: 23758600 |
| [12] |
Klingenberg CP, Barluenga M, Meyer A. Shape analysis of symmetric structures: quantifying variation among individuals and asymmetry[J]. Evolution, 2002,56(10):1909-1920.
doi: 10.1111/j.0014-3820.2002.tb00117.x pmid: 12449478 |
| [13] |
Du S, Xu Y, Wan T, et al. Robust iterative closest point algorithm based on global reference point for rotation invariant registration[J]. PLoS One, 2017,12(11):e0188039.
doi: 10.1371/journal.pone.0188039 pmid: 29176780 |
| [14] |
Damstra J, Fourie Z, De Wit M, et al. A three-dimensional comparison of a morphometric and conventional cephalometric mid-sagittal planes for craniofacial asymmetry[J]. Clin Oral Investig, 2012,16(1):285-294.
doi: 10.1007/s00784-011-0512-4 pmid: 21271348 |
| [15] |
Besl PJ, McKay ND. A method for registration of 3-D shapes[J]. IEEE T Pattern Anal, 1992,14(2):239-256.
doi: 10.1109/34.121791 |
| [16] |
Verhoeven TJ, Coppen C, Barkhuysen R, et al. Three dimensio-nal evaluation of facial asymmetry after mandibular reconstruction: validation of a new method using stereophotogrammetry[J]. Int J Oral Maxillofac Surg, 2013,42(1):19-25.
doi: 10.1016/j.ijom.2012.05.036 pmid: 22939875 |
| [17] |
De Momi E, Chapuis J, Pappas I, et al. Automatic extraction of the mid-facial plane for cranio-maxillofacial surgery planning[J]. Int J Oral Maxillofac Surg, 2006,35(7):636-642.
doi: 10.1016/j.ijom.2006.01.028 pmid: 16542822 |
| [18] |
Xiong Y, Zhao Y, Yang H, et al. Comparison between interactive closest point and procrustes analysis for determining the median sagittal plane of three-dimensional facial data[J]. J Craniofac Surg, 2016,27(2):441-444.
doi: 10.1097/SCS.0000000000002376 pmid: 26825747 |
| [19] |
Richtsmeier JT, Lele S. A coordinate-free approach to the analysis of growth patterns: models and theoretical considerations[J]. Biol Rev Camb Philos Soc, 1993,68(3):381-411.
doi: 10.1111/j.1469-185x.1993.tb00737.x pmid: 8347767 |
| [20] |
Lele S, Richtsmeier JT. Euclidean distance matrix analysis: a coordinate-free approach for comparing biological shapes using landmark data[J]. Am J Phys Anthropol, 1991,86(3):415-421.
doi: 10.1002/ajpa.1330860307 pmid: 1746646 |
| [21] |
Verhoeven TJ, Nolte JW, Maal TJ, et al. Unilateral condylar hyperplasia: a 3-dimensional quantification of asymmetry[J]. PLoS One, 2013,8(3):e59391.
doi: 10.1371/journal.pone.0059391 pmid: 23544063 |
| [22] |
Gateno J, Jajoo A, Nicol M, et al. The primal sagittal plane of the head: a new concept[J]. Int J Oral Maxillofac Surg, 2016,45(3):399-405.
doi: 10.1016/j.ijom.2015.11.013 pmid: 26708049 |
| [23] |
Chetverikov D, Stepanov D, Krsek P. Robust Euclidean alignment of 3D point sets: the trimmed iterative closest point algorithm[J]. Image Vision Comput, 2005,23(3):299-309.
doi: 10.1016/j.imavis.2004.05.007 |
| [24] | Zelditch ML, Swiderski DL, Sheets DH, et al. Geometric morphometrics for biologists: A primer [M]. San Diego: Elsevier Academic Press, 2004: 293-319. |
| [25] |
Walters M, Claes P, Kakulas E, et al. Robust and regional 3D facial asymmetry assessment in hemimandibular hyperplasia and hemimandibular elongation anomalies[J]. Int J Oral Maxillofac Surg, 2013,42(1):36-42.
doi: 10.1016/j.ijom.2012.05.021 pmid: 22749574 |
| [26] |
Claes P, Walters M, Clement J. Improved facial outcome assessment using a 3D anthropometric mask[J]. Int J Oral Maxillofac Surg, 2012,41(3):324-330.
doi: 10.1016/j.ijom.2011.10.019 pmid: 22103995 |
| [27] |
Claes P, Walters M, Vandermeulen D, et al. Spatially-dense 3D facial asymmetry assessment in both typical and disordered growth[J]. J Anat, 2011,219(4):444-455.
doi: 10.1111/j.1469-7580.2011.01411.x |
| [28] |
Wu J, Heike C, Birgfeld C, et al. Measuring symmetry in children with unrepaired cleft lip: defining a standard for the three-dimensional midfacial reference plane[J]. Cleft Palate Craniofac J, 2016,53(6):695-704.
doi: 10.1597/15-053 pmid: 26752127 |
| [29] |
Perlyn CA, DeLeon VB, Babbs C, et al. The craniofacial phenotype of the Crouzon mouse: analysis of a model for syndromic craniosynostosis using three-dimensional MicroCT[J]. Cleft Palate Craniofac J, 2006,43(6):740-748.
doi: 10.1597/05-212 pmid: 17105336 |
| [30] |
Richtsmeier JT, Cole TR, Krovitz G, et al. Preoperative mor-phology and development in sagittal synostosis[J]. J Craniofac Genet Dev Biol, 1998,18(2):64-78.
pmid: 9672839 |
| [31] |
Richtsmeier JT, Lele S. Analysis of craniofacial growth in Crouzon syndrome using landmark data[J]. J Craniofac Genet Dev Biol, 1990,10(1):39-62.
pmid: 2373755 |
| [32] |
Dufresne C, Richtsmeier JT. Interaction of craniofacial dysmorphology, growth, and prediction of surgical outcome[J]. J Craniofac Surg, 1995,6(4):270-281.
doi: 10.1097/00001665-199507000-00003 pmid: 9020701 |
| [33] | Ferrario VF, Sforza C, Pizzini G, et al. Sexual dimorphism in the human face assessed by Euclidean distance matrix analysis[J]. J Anat, 1993,183(Pt 3):593-600. |
| [34] |
Ferrario VF, Sforza C, Miani AJ, et al. Maxillary versus mandi-bular arch form differences in human permanent dentition assessed by Euclidean-distance matrix analysis[J]. Arch Oral Biol, 1994,39(2):135-139.
doi: 10.1016/0003-9969(94)90108-2 pmid: 8185498 |
| [35] |
Ferrario VF, Sforza C, Miani AJ, et al. Human dental arch shape evaluated by Euclidean-distance matrix analysis[J]. Am J Phys Anthropol, 1993,90(4):445-453.
doi: 10.1002/ajpa.1330900405 pmid: 8476003 |
| [36] |
Ferrario VF, Sforza C, Miani AJ, et al. Dental arch asymmetry in young healthy human subjects evaluated by Euclidean distance matrix analysis[J]. Arch Oral Biol, 1993,38(3):189-194.
doi: 10.1016/0003-9969(93)90027-j pmid: 8489412 |
| [37] | 聂琼, 林久祥. Angle Ⅱ1与正常牙合牙弓形态差异——应用欧几里德距离矩阵分析法[J]. 实用口腔医学杂志, 2005,21(5):659-662. |
| [1] | QIU Shu-ting,ZHU Yu-jia,WANG Shi-min,WANG Fei-long,YE Hong-qiang,ZHAO Yi-jiao,LIU Yun-song,WANG Yong,ZHOU Yong-sheng. Preliminary clinical application verification of complete digital workflow of design lips symmetry reference plane based on posed smile [J]. Journal of Peking University (Health Sciences), 2022, 54(1): 193-199. |
| [2] | ZHU Yu-jia,XU Qing,ZHAO Yi-jiao,ZHANG Lei,FU Zi-wang,WEN Ao-nan,GAO Zi-xiang,ZHANG Jun,FU Xiang-ling,WANG Yong. Deep learning-assisted construction of three-demensional facial midsagittal plane [J]. Journal of Peking University (Health Sciences), 2022, 54(1): 134-139. |
| [3] | XIONG Yu-Xue, Yang-Hui-Fang, Zhao-Yi-Jiao, WANG Yong. Comparison of two kinds of methods evaluating the degree of facial asymmetry by three-dimensional data [J]. Journal of Peking University(Health Sciences), 2015, 47(2): 340-343. |
|
||