北京大学学报(医学版) ›› 2019, Vol. 51 ›› Issue (6): 1130-1137. doi: 10.19723/j.issn.1671-167X.2019.06.027
个性化根形种植体的螺纹形态对周围牙槽骨应力分布影响的三维有限元分析
林春平1,卢松鹤2,朱浚鑫2,胡洪成2,岳兆国2,唐志辉2,△( )
)
- 1. 北京大学口腔医学院·口腔医院,牙周科 口腔数字化医疗技术和材料国家工程实验室 口腔数字医学北京市重点实验室, 北京 100081
2. 北京大学口腔医学院·口腔医院第二门诊部, 北京 100101
Influence of thread shapes of custom-made root-analogue implants on stress distribution of peri-implant bone: A three-dimensional finite element analysis
Chun-ping LIN1,Song-he LU2,Jun-xin ZHU2,Hong-cheng HU2,Zhao-guo YUE2,Zhi-hui TANG2,△( )
)
- 1. Department of Periodontitis, Peking University School and Hospital of Stomatology & National Engineering Laboratory for Digital and Material Technology of Stomatology & Beijing Key Laboratory of Digital Stomatology, Beijing 100081, China
2. Second Clinical Division, Peking University School and Hospital of Stomatology, Beijing 100101, China
摘要:
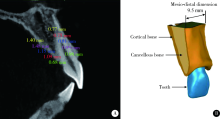
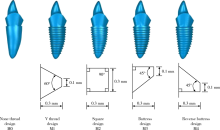
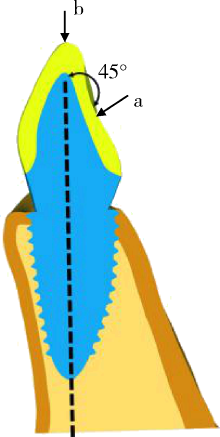
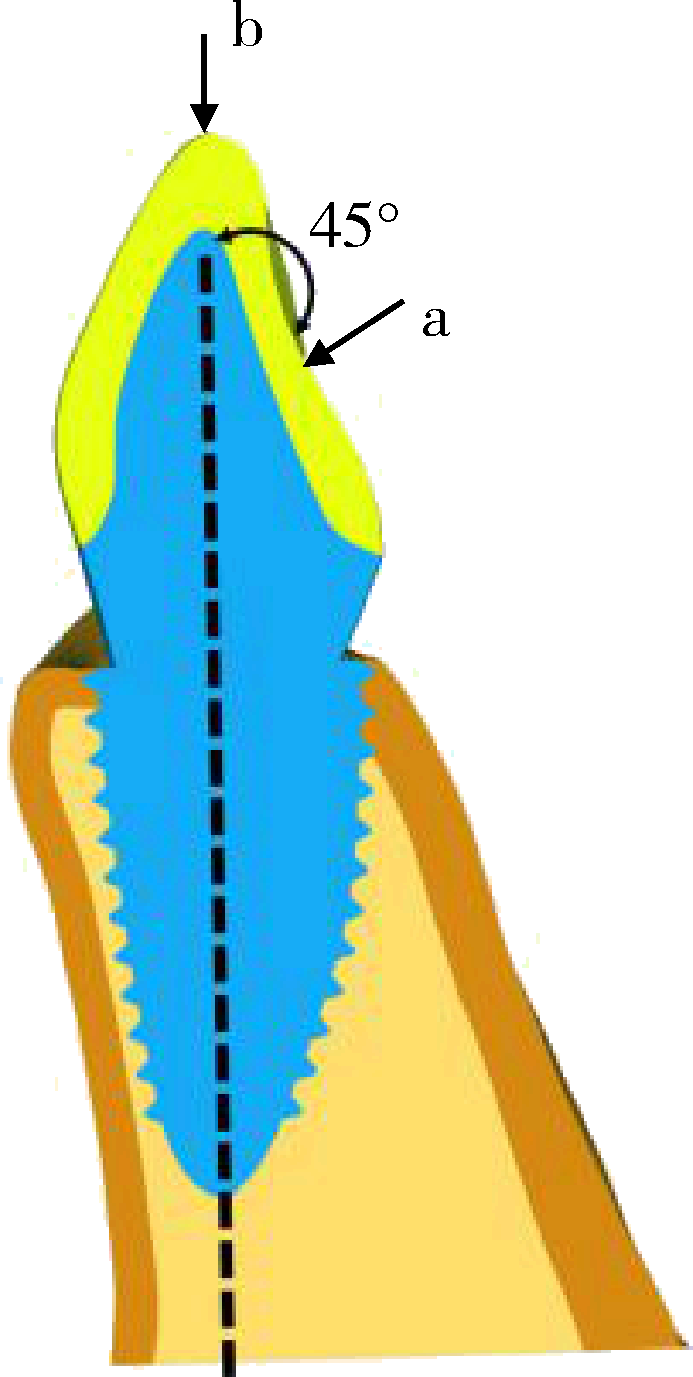
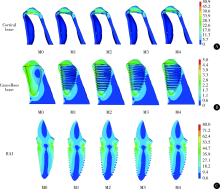
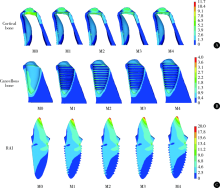
目的 探讨个性化根形种植体的螺纹形态设计对周围牙槽骨应力分布的影响。方法 通过逆向建模技术建立带有矩形、V形、支撑形、反支撑形螺纹和不带螺纹的一段式个性化根形牙种植体的三维有限元模型,分别加载与种植体长轴呈45°角及0°角的100 N的力,导入Ansys 16软件计算不同螺纹个性化根形种植体周围骨组织von Mises应力分布的情况。结果 倾斜45°角加载时,皮质骨内应力主要集中于种植体颈部周缘及螺纹顶端,松质骨内应力主要分布于种植体唇侧颈部、螺纹顶端和植体根尖部。垂直(0°角)加载时,皮质骨内应力主要集中于种植体颈部处,松质骨内应力主要分布于根尖部以及唇侧根下部。加载时,螺纹组相对无螺纹组应力分布更加均匀,各螺纹组间无明显差别。松质骨内各螺纹植体应力主要集中在螺纹顶端处,根尖处的应力集中较少。与矩形螺纹相比,V形、支撑形和反支撑形种植体在松质骨内的应力分布更为均匀。结论 螺纹设计可以优化个性化根形种植体周围皮质骨和松质骨内的应力分布,减小皮质骨内的应力集中,V形、支撑形、反支撑形相比矩形螺纹设计,应力分布更加均匀。
中图分类号:
- R783.6
| [1] | Kohal RJ, Hürzeler MB, Mota LF , et al. Custom-made root analogue titanium implants placed into extraction sockets: An experimental study in monkeys[J]. Clin Oral Implants Res, 1997,8(5):386-392. |
| [2] | Mangano FG, de Franco M, Caprioglio A , et al. Immediate, non-submerged, root-analogue direct laser metal sintering (DLMS) implants: a 1-year prospective study on 15 patients[J]. Lasers Med Sci, 2014,29(4):1321-1328. |
| [3] | Nair A, Prithviraj DR, Regish KM , et al. Custom milled zirconia implant supporting an ceramic zirconia restoration: a clinical report[J]. KUMU, 2013,11(44):328-331. |
| [4] | Hodosh M, Povar M, Shklar G , et al. The dental polymer implant concept[J]. J Prosthet Dent, 1969,22(3):371-380. |
| [5] | Lundgren D, Rylander H, Andersson M , et al. Healing-in of root analogue titanium implants placed in extraction sockets: an experimental study in the beagle dog[J]. Clin Oral Implants Res, 1992,3(3):136-143. |
| [6] | Pirker W, Kocher A . Immediate, non-submerged, root-analogue zirconia implant in single tooth replacement[J]. Int J Oral Maxillofac Surg, 2008,37(3):293-295. |
| [7] | Mangano FG, Cirotti B, Sammons RL , et al. Custom-made, root-analogue direct laser metal forming implant: a case report[J]. Lasers Med Sci, 2012,27(6):1241-1245. |
| [8] | Figliuzzi M, Mangano F, Mangano C . A novel root analogue den-tal implant using CT scan and CAD/CAM: selective laser melting technology[J]. Int J Oral Maxillofac Surg, 2012,41(7):858-862. |
| [9] | Ivanoff CJ, Grondahl K, Sennerby L , et al. Influence of variations in implant diametes:a 3-to 5-year retrospective clinical report[J]. Int J Oral Maxillofac Implants, 1999,14(2):173-180. |
| [10] | Hansson S, Werke M . The implant thread as a retention element in cortical bone: the effect of thread size and thread profile: a finite element study[J]. J Biomech, 2003,36(9):1247-1258. |
| [11] | Huang HL, Chang CH, Hsu JT , et al. Comparison of implant body designs and threaded designs of dental implants: a 3-dimensional finite element analysis[J]. Int J Oral Maxillofac Implants, 2007,22(4):551-562. |
| [12] | Huang HL, Hsu JT, Fuh LJ , et al. Bone stress and interfacial sliding analysis of implant designs on an immediately loaded maxillary implant: a non-linear finite element study[J]. J Dent, 2008,36(6):409-417. |
| [13] | Cehreli M, Duyck J, de Cooman M , et al. Implant design and interface force transfer. A photoelastic and strain-gauge analysis[J]. Clin Oral Impl Res, 2004,15(2):249-257. |
| [14] | 梅双, 董福生, 董玉英 , 等. 种植体不同螺纹底部形态对骨界面应力分布的影响[J]. 现代口腔医学杂志, 2016,30(3):138-141. |
| [15] | Karoussis IK, Bragger U, Salvi GE , et al. Effect of impalnt design on survival and success rates of titanium oral implants: a 10-year prospective cohort study of the ITI Dental Implant System[J]. Clin Oral Implants Res, 2004,15(1):8-17. |
| [16] | Anssari Moin D, Hassan B, Wismeijer D . A patient specific biomechanical analysis of custom root analogue implant designs on alveolar bone stress: A finite element study[J]. Int J Dent, 2016,8242535. doi: 10.1155/2016/8242535. |
| [17] | Chen J, Zhang Z, Chen X , et al. Influence of custom-made implant designs on the biomechanical performance for the case of immediate post-extraction placement in the maxillary esthetic zone: a finite element analysis[J]. Comput Methods Biomech Biomed Engin, 2017,20(6):636-644. |
| [18] | 孔亮, 刘宝林, 李德华 , 等. 不同螺纹形态种植体对颌骨应力影响的三维有限元比较研究[J]. 口腔医学研究, 2006,22(2):155-158. |
| [19] | Tribst JPM, de Morais DC, Alonso AA , et al. Comparative three-dimensional finite element analysis of implant-supported fixed complete arch mandibular prostheses in two materials[J]. J Indian Prosthodont Soc, 2017,17(3):255-260. |
| [20] | Alvarez-Arenal A, Gonzalez-Gonzalez I, Dellanos-Lanchares H , et al. Influence of implant positions and occlusal forces on peri-implant bone stress in mandibular two-implant overdentures: a 3D finite element analysis[J]. J Oral Implantol, 2017,43(6):419-428. |
| [21] | Sugiura T, Yamamoto K, Horita S , et al. Effects of implant tilting and the loading direction on the displacement and micromotion of immediately loaded implants: an in vitro experiment and finite element analysis[J]. J Periodontal Implant Sci, 2017,47(4):251-262. |
| [22] | Yamanishi Y, Yamaguchi S, Imazato S , et al. Influences of implant neck design and implant-abutment joint type on peri-implant bone stress and abutment micromovement: three-dimensional finite element analysis[J]. Dent Mater, 2012,28(11):1126-1133. |
| [23] | Toniollo MB, Macedo AP, Pupim D , et al. Finite element analysis of bone stress in the posterior mandible using regular and short implants, in the same context, with splinted and nonsplinted prostheses[J]. Int J Oral Maxillofac Implants, 2017,32(4):e199-e206. |
| [24] | Choi JH, Lee BY, Han JS . Boundary integral method for shape optimization of interface problems and its application to implant design in dentistry[J]. Comput Methods Appl Mech Eng, 2001,190(51-52):6906-6926. |
| [25] | Mosavar A, Ziaei A, Kadkhodaei M . The effect of implant thread design on stress distribution in anisotropic bone with different osseointegration conditions: a finite element analysis[J]. Int J Oral Maxillofac Implants, 2015,30(6):1317-1326. |
| [26] | Misch CE . Dental implant prosthetics[M]. St Louis, MO: Mosby, 2005: 322-347. |
| [27] | Bumgardner JD, Boring JG, Cooper RC Jr , et al. Preliminary evaluation of a new dental implant design in canine models[J]. Implant Dent, 2000,9(3):252-260. |
| [28] | Steigenga J, Al-Shammari K, Misch C , et al. Effects of implant thread geometry on percentage of osseointegration and resistance to reverse torque in the tibia of rabbits[J]. J Periodontol, 2004,75(9):1233-1241. |
| [29] | Eraslan O, Inan O . The effect of thread design on stress distribution in a solid screw implant: a 3D finite element analysis[J]. Clin Oral Investig, 2010,14(4):411-416. |
| [30] | Misch CE . Scientific rationale fordental implant design[M]// Misch CE. Contemporary Implant Dentistry. St. Louis, MO: Elsevier Mosby, 2008: 200-232. |
| [31] | Javed F, Romanos GE . The role of primary stability for successful immediate loading of dental implants. A literature review[J]. J Dent, 2010,38(8):612-620. |
| [32] | Baggi L, Di Girolamo M, Vairo G , et al. Comparative evaluation of osseointegrated dental implants based on platform-switching concept: influence of diameter, length, thread shape, and in-bone positioning depth on stress-based performance[J]. Comput Math Methods Med, 2013,250929. doi: 10.1155/2013/250929. |
| [33] | Oswal MM, Amasi UN, Oswal MS , et al. Influence of three diffe-rent implant thread designs on stress distribution: A three-dimensional finite element analysis[J]. J Indian Prosthodont Soc, 2016,16(4):359-365. |
| [34] | Ding X, Zhu XH, Liao SH , et al. Implant-bone interface stress distribution in immediately loaded implants of different diameters: a three-dimensional finite element analysis[J]. J Prosthodont, 2009,18(5):393-402. |
| [35] | Chang PK, Chen YC, Huang CC , et al. Distribution of micromotion in implants and alveolar bone with different thread profiles in immediate loading: a finite element study[J]. Int J Oral Maxillofac Implants, 2012,27(6):e96-101. |
| [36] | Fuh LJ, Hsu JT, Huang HL , et al. Biomechanical investigation of thread designs and interface conditions of zirconia and titanium dental implants with bone: three-dimensional numeric analysis[J]. Int J Oral Maxillofac Implants, 2013,28(2):e64-71. |
| [37] | Steigenga JT, al-Shammari KF, Nociti FH , et al. Dental implant design and its relationship to long-term implant success[J]. Implant Dent, 2003,12(4):306-317. |
| [1] | 王聪伟,高敏,于尧,章文博,彭歆. 游离腓骨瓣修复下颌骨缺损术后义齿修复的临床分析[J]. 北京大学学报(医学版), 2024, 56(1): 66-73. |
| [2] | 丁茜,李文锦,孙丰博,谷景华,林元华,张磊. 表面处理对氧化钇和氧化镁稳定的氧化锆种植体晶相及断裂强度的影响[J]. 北京大学学报(医学版), 2023, 55(4): 721-728. |
| [3] | 欧蒙恩,丁云,唐卫峰,周永胜. 基台边缘-牙冠的平台转移结构中粘接剂流动的三维有限元分析[J]. 北京大学学报(医学版), 2023, 55(3): 548-552. |
| [4] | 孙菲,刘建,李思琪,危伊萍,胡文杰,王翠. 种植体黏膜下微生物在健康种植体和种植体周炎中的构成与差异:一项横断面研究[J]. 北京大学学报(医学版), 2023, 55(1): 30-37. |
| [5] | 马珂楠,陈虎,沈妍汝,周永胜,王勇,孙玉春. 选择性激光熔化打印可摘局部义齿圆环形卡环固位力的有限元分析[J]. 北京大学学报(医学版), 2022, 54(1): 105-112. |
| [6] | 任爽,时会娟,张家豪,刘振龙,邵嘉艺,朱敬先,胡晓青,黄红拾,敖英芳. 前交叉韧带重建术后移植物应力的有限元分析[J]. 北京大学学报(医学版), 2021, 53(5): 865-870. |
| [7] | 梁峰,吴敏节,邹立东. 后牙区单牙种植修复5年后的临床修复疗效观察[J]. 北京大学学报(医学版), 2021, 53(5): 970-976. |
| [8] | 周伟,安金刚,荣起国,张益. 下颌骨颏部骨折联合双侧髁突囊内骨折致伤机制的三维有限元分析[J]. 北京大学学报(医学版), 2021, 53(5): 983-989. |
| [9] | 姜又升,冯琳,高学军. 垫底材料弹性模量对髓腔固位冠修复后上颌前磨牙应力分布的影响[J]. 北京大学学报(医学版), 2021, 53(4): 764-769. |
| [10] | 释栋,曹婕,戴世爱,孟焕新. 植体周炎再生治疗短期疗效观察[J]. 北京大学学报(医学版), 2020, 52(1): 58-63. |
| [11] | 付宏宇,王芳芳,侯晓玫. 控制记忆合金丝镍钛根管锉弯曲性能有限元分析模型的构建及力学分析[J]. 北京大学学报(医学版), 2019, 51(1): 131-135. |
| [12] | 刘潇倩,陈秋雯,冯海兰,王兵,屈健,孙振,衡墨迪,潘韶霞. 无牙颌患者locator附着体种植覆盖义齿修复后口腔卫生维护的纵向研究[J]. 北京大学学报(医学版), 2019, 51(1): 136-144. |
| [13] | 杨殷杰,侯本祥,侯晓玫. 高压蒸汽灭菌对R-相镍钛锉表面形态及疲劳折断性能的影响[J]. 北京大学学报(医学版), 2018, 50(5): 882-886. |
| [14] | 吴敏节,邹立东,梁峰. 上前牙即刻种植即刻修复负载3年后软、硬组织变化的临床观察[J]. 北京大学学报(医学版), 2018, 50(4): 694-699. |
| [15] | 刘婧寅,陈飞,葛严军,魏菱,潘韶霞,冯海兰. 选择性激光熔化种植体对早期骨矿化沉积率的影响[J]. 北京大学学报(医学版), 2018, 50(1): 117-122. |
|
||